
The tangent lines of the nine-point circle at the midpoints of the sides of △ ABC are parallel to the sides of the orthic triangle, forming a triangle similar to the orthic triangle. The orthic triangle of an acute triangle gives a triangular light route. The sides of the orthic triangle are parallel to the tangents to the circumcircle at the original triangle's vertices. This is the solution to Fagnano's problem, posed in 1775. In any acute triangle, the inscribed triangle with the smallest perimeter is the orthic triangle. The extended sides of the orthic triangle meet the opposite extended sides of its reference triangle at three collinear points. Let A, B, C denote the vertices and also the angles of the triangle, and let a = | B C ¯ |, b = | C A ¯ |, c = | A B ¯ | If one angle is a right angle, the orthocenter coincides with the vertex at the right angle. and determining when three lengths form a triangle Notes and practice worksheet that identify the. The orthocenter lies inside the triangle if and only if the triangle is acute. The three (possibly extended) altitudes intersect in a single point, called the orthocenter of the triangle, usually denoted by H. Three altitudes intersecting at the orthocenter

It is common to mark the altitude with the letter h (as in height), often subscripted with the name of the side the altitude is drawn to. How to Find Altitude in Geometry : Math Fundamentals eHowEducation.
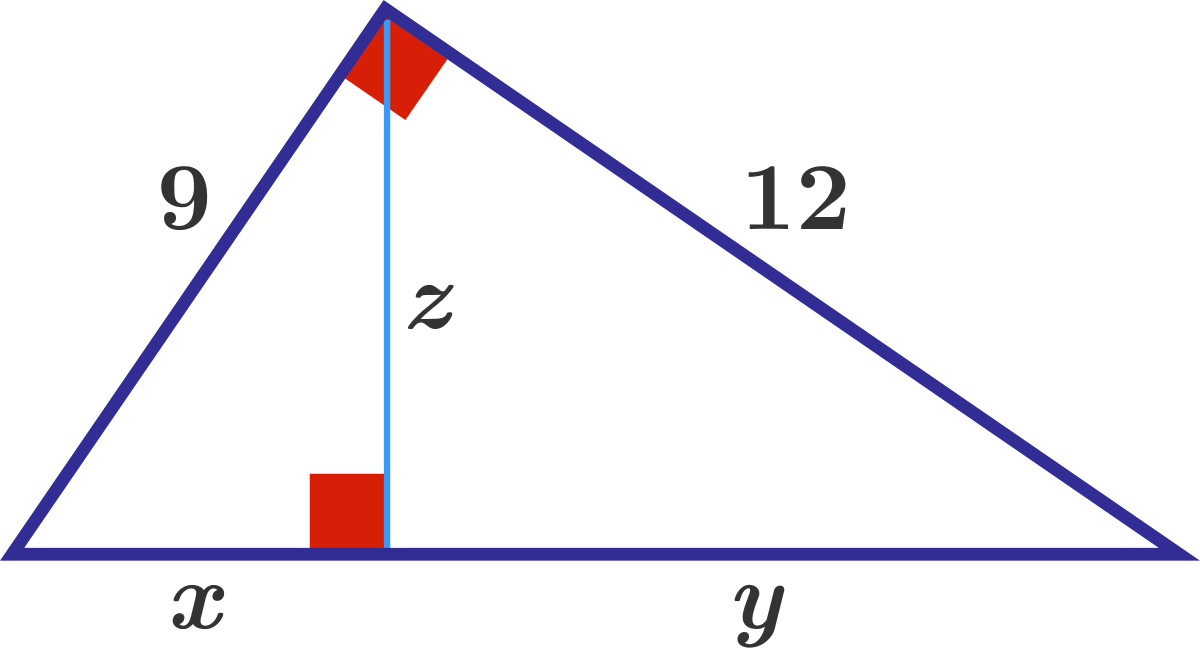
If the optional argument H is given, the object returned ( hA ) is a line segment AH where H is the projection of A onto the side BC. Also the altitude having the incongruent side as its base will be the angle bisector of the vertex angle. An altitude from the vertex A of a triangle ABC is a line segment (or its extension) from vertex A perpendicular to the side BC. In an isosceles triangle (a triangle with two congruent sides), the altitude having the incongruent side as its base will have the midpoint of that side as its foot. The altitudes are also related to the sides of the triangle through the trigonometric functions. Thus, the longest altitude is perpendicular to the shortest side of the triangle. It is a special case of orthogonal projection.Īltitudes can be used in the computation of the area of a triangle: one-half of the product of an altitude's length and its base's length equals the triangle's area. The process of drawing the altitude from the vertex to the foot is known as dropping the altitude at that vertex. A segment that connects the vertex and the opposite side at 90 degrees.

A segment that connects two midpoints of two sides. A segment with endpoints at the vertex and midpoint of the opposite side. An altitude of a triangle is a perpendicular segment from a vertex to the line. Which of the following describes a median of a triangle A segment that passes through the middle of the triangle. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. Essential Question: How can you find the balance point or center of. The intersection of the extended base and the altitude is called the foot of the altitude. This line containing the opposite side is called the extended base of the altitude. In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to (i.e., forming a right angle with) a line containing the base (the side opposite the vertex). Substitute for one variable and combine to one equation with one unknown.The three altitudes of a triangle intersect at the orthocenter, which for an acute triangle is inside the triangle. Right Triangle Altitude Theorem: The altitude from the right angle vertex to the hypotenuse is equal to the geometric mean of the two segments of the hypotenuse.

#QUESTIONS ON FINDING ALTITUDE GEOMETRY FREE#
They aren't linear so they may not satisfy the simple " $k$ (linear and independant) equations $k$ unknowns = solvable" mantra, but they probably do. Improve your math knowledge with free questions in Identify medians, altitudes, angle bisectors, and perpendicular bisectors and thousands of other math. (and one assumption that all terms are positive)


 0 kommentar(er)
0 kommentar(er)
